nLab Knizhnik-Zamolodchikov equation

under construction

Idea

General

Given a suitable Lie algebra $\mathfrak{g}$ a Knizhnik-Zamolodchikov equation is the equation expressing flatness of certain class of vector bundles with connection on the configuration space of points of $N$ distinct points in the plane $\mathbb{C}$. It appeared in the study of Wess-Zumino-Novikov-Witten model (WZNW model) of 2d CFT in (Knizhnik-Zamolodchikov 84).

The Knizhnik-Zamolodchikov equation involves what is called the Knizhnik-Zamolodchikov connection and it is related to monodromy representations of Artin’s braid group.

In the standard variant, the basic data involves a given complex simple Lie algebra $\mathfrak{g}$ with a fixed bilinear invariant polynomial $(-,-)$ (the Killing form) and $N$ (not necessarily finite-dimensional) representations $V_1,\ldots, V_n$ of $\mathfrak{g}$. Let $V \,=\, V_1 \otimes \ldots \otimes V_N$.

(…)

From geometric quantization of Chern-Simons theory

The existence of the Knizhnik-Zamolodchikov connection can naturally be understood from the holographic quantization of the WZW model on the Lie group $G$ by geometric quantization of $G$-Chern-Simons theory:

As discussed there, for a 2-dimensional manifold $\Sigma$, a choice of polarization of the phase space of 3d Chern-Simons theory on $\Sigma$ is naturally induced by a choice $J$ of conformal structure on $\Sigma$. Once such a choice is made, the resulting space of quantum states $\mathcal{H}_\Sigma^{(J)}$ of the Chern-Simons theory over $\Sigma$ is naturally identified with the space of conformal blocks of the WZW model 2d CFT on the Riemann surface $(\Sigma, J)$.

But since, from the point of view of the 3d Chern-Simons theory, the polarization $J$ is an arbitrary choice, the space of quantum states $\mathcal{H}_\Sigma^{(J)}$ should not depend on this choice, up to specified equivalence. Formally this means that as $J$ varies (over the moduli space of conformal structures on $\Sigma$) the $\mathcal{H}_{\Sigma}^{(J)}$ should form a vector bundle on this moduli space of conformal structures which is equipped with a flat connection whose parallel transport hence provides equivalences between between the fibers $\mathcal{H}_{\Sigma}^{(J)}$ of this vector bundle.

This flat connection is the Knizhnik-Zamolodchikov connection. This was maybe first realized and explained in (Witten 89).

Definition

For the Definition of the Knizhnik-Zamolodchikov connection we need the following notation:

1. configuration spaces of points

   For $N_{\mathrm{f}} \in \mathbb{N}$ write

   (1)$$\underset{{}^{\{1,\cdots,N_{\mathrm{f}}\}}}{Conf}(\mathbb{R}^2) \;\coloneqq\; (\mathbb{R}^2)^n \backslash FatDiagonal$$

   for the ordered configuration space of n points in the plane, regarded as a smooth manifold.

   Identifying the plane with the complex plane $\mathbb{C}$, we have canonical holomorphic coordinate functions

   (2)$$(z_1, \cdots, z_{N_{\mathrm{f}}}) \;\colon\; \underset{{}^{\{1,\cdots,n\}}}{Conf}(\mathbb{R}^2) \longrightarrow \mathbb{C}^{N_{\mathrm{f}}} \,.$$

2. horizontal chord diagrams

   (3)$$\mathcal{A}^{{}^{pb}}_{N_{\mathrm{f}}} \;\coloneqq\; Span\big(\mathcal{D}^{{}^{pb}}_{N_{\mathrm{f}}}\big)/4T$$

   for the quotient vector space of the linear span of horizontal chord diagrams on $n$ strands by the 4T relations (infinitesimal braid relations), regarded as an associative algebra under concatenation of strands (here).

Definition

(Knizhnik-Zamolodchikov form)

The universal Knizhnik-Zamolodchikov form is the horizontal chord diagram-algebra valued differential form (3) on the configuration space of points (1)

(4)$$\omega_{KZ} \;\in\; \Omega \big( \underset{{}^{\{1,\cdots,N_{\mathrm{f}}\}}}{Conf}(\mathbb{C}) \,, \mathcal{A}^{{}^{pb}}_{N_{\mathrm{f}}} \big)$$

given in the canonical coordinates (2) by:

(5)$$\omega_{KZ} \;\coloneqq\; \underset{ i \lt j \in \{1, \cdots, n\} }{\sum} d_{dR} log\big( z_i - z_j \big) \otimes t_{i j} \,,$$

where

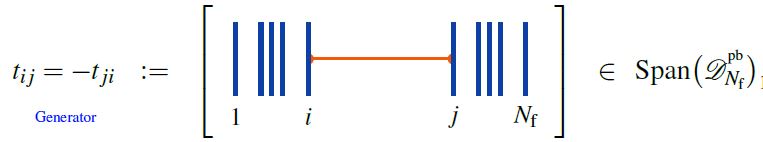
is the horizontal chord diagram with exactly one chord, which stretches between the $i$th and the $j$th strand.

Regarded as a connection form for a connection on a vector bundle, this defines the universal Knizhnik-Zamolodchikov connection $\nabla_{KZ}$, with covariant derivative

$$\nabla \phi \;\coloneqq\; d \phi + \omega_{KV} \wedge \phi$$

for any smooth function

$$\phi \;\colon\; \underset{{}^{\{1,\cdots,N_{\mathrm{f}}\}}}{Conf}(\mathbb{C}) \longrightarrow \mathcal{A}^{{}^{pb}}_{N_{\mathrm{f}}} Mod$$

with values in modules over the algebra of horizontal chord diagrams modulo 4T relations.

The condition of covariant constancy

$$\nabla_{KZ} \phi \;=\; 0$$

is called the Knizhnik-Zamolodchikov equation.

Finally, given a metric Lie algebra $\mathfrak{g}$ and a tuple of Lie algebra representations

$$( V_1, \cdots, V_{N_{\mathrm{f}}} ) \;\in\; (\mathfrak{g} Rep_{/\sim})^{N_{\mathrm{f}}} \,,$$

the corresponding endomorphism-valued Lie algebra weight system

$$w_{V} \;\colon\; \mathcal{A}^{{}^{pf}}_{N_{\mathrm{f}}} \longrightarrow End_{\mathfrak{g}}\big( V_1 \otimes \cdots V_{N_{\mathrm{f}}} \big)$$

turns the universal Knizhnik-Zamolodchikov form (4) into a endomorphism ring-valued differential form

(6)$$\omega_{KZ} \;\coloneqq\; \underset{ i \lt j \in \{1, \cdots, n\} }{\sum} d_{dR} log\big( z_i - z_j \big) \otimes w_V(t_{i j}) \;\in\; \Omega \big( \underset{{}^{\{1,\cdots,N_{\mathrm{f}}\}}}{Conf}(\mathbb{C}) \,, End\big(V_1 \otimes \cdot V_{N_{\mathrm{f}}} \big) \big) \,.$$

The universal formulation (4) is highlighted for instance in Bat-Natan 95, Section 4.2, Lescop 00, p. 7. Most authors state the version after evaluation in a Lie algebra weight system, e.g. Kohno 14, Section 5.

Proposition

(Knizhnik-Zamolodchikov connection is flat)

The Knizhnik-Zamolodchikov connection $\omega_{ZK}$ (Def. ) is flat:

$$d \omega_{ZK} + \omega_{ZK} \wedge \omega_{ZK} \;=\; 0 \,.$$

Proposition

(Kontsevich integral for braids)

The Dyson formula for the holonomy of the Knizhnik-Zamolodchikov connection (Def. ) is called the Kontsevich integral on braids.

(e.g. Lescop 00, side-remark 1.14)

Related entries

• Kohno-Drinfeld theorem

• Hitchin connection

• Pochhammer loop

References

General

The original articles:

• Vadim Knizhnik, Alexander Zamolodchikov, Current algebra and Wess–Zumino model in two-dimensions, Nucl. Phys. B247, 83–103 (1984) doi, MR87h:81129

• Alexander Belavin, Alexander Polyakov, Alexander Zamolodchikov, Infinite conformal symmetry in two-dimensional quantum field theory (1984) Nucl. Phys. B 241 (2): 333–80.

• Daniel Friedan, Stephen Shenker, The analytic geometry of two-dimensional conformal field theory, Nuclear Physics B281 (1987) (pdf)

Textbook account, specifically for the $\mathfrak{su}(2)$/$\mathfrak{sl}(2,\mathbb{Z})$-WZW model:

• Philippe Di Francesco, Pierre Mathieu, David Sénéchal: §15.3.2, §15.4 in: Conformal field theory, Springer (1997) [doi:10.1007/978-1-4612-2256-9]

Relation to braid representations:

• Ivan Todorov, Ludmil Hadjiivanov, Monodromy Representations of the Braid Group, Phys. Atom. Nucl. 64 (2001) 2059-2068; Yad.Fiz. 64 (2001) 2149-2158 [arXiv:hep-th/0012099, doi:10.1134/1.1432899]

• Ivan Marin, Sur les représentations de Krammer génériques, Annales de l’Institut Fourier, 57 6 (2007) 1883-1925 [numdam:AIF_2007__57_6_1883_0]

• Anton Alekseev, Florian Naef, Muze Ren. Generalized Pentagon Equations (2024). (arXiv:2402.19138).

and understood in terms of anyon statistics:

• Xia Gu, Babak Haghighat, Yihua Liu, Ising- and Fibonacci-Anyons from KZ-equations, J. High Energ. Phys. 2022 15 (2022) [arXiv:2112.07195, doi:10.1007/JHEP09(2022)015]

The generalization to higher genus surfaces:

• D. Bernard, On the Wess-Zumino-Witten models on the torus, Nucl. Phys. B 303 77-93 (1988)

• D. Bernard, On the Wess-Zumino-Witten models on Riemann surfaces, Nucl. Phys. B 309 145-174 (1988)

The interpretation of this connection in terms of the geometric quantization of Chern-Simons theory/Wess-Zumino-Witten model:

• Edward Witten, p. 20 of: Quantum Field Theory and the Jones Polynomial, Commun. Math. Phys. 121 3 (1989) 351–399. $[$euclid.cmp/1104178138, MR0990772$]$

Review:

• Ivan Cherednik, Lectures on Knizhnik-Zamolodchikov equations and Hecke algebras, Mathematical Society of Japan Memoirs 1998 (1998) 1-96 $[$doi:10.2969/msjmemoirs/00101C010$]$

  (in relation to Hecke algebras)

• Toshitake Kohno, §1.5 and §2.1 in: Conformal field theory and topology, transl. from the 1998 Japanese original by the author. Translations of Mathematical Monographs 210. Iwanami Series in Modern Mathematics. Amer. Math. Soc. 2002 $[$AMS:mmono-210$]$

  (relating to conformal blocks and braid representations)

• Ralph Blumenhagen, Erik Plauschinn, §3.5 of: Introduction to Conformal Field Theory – With Applications to String Theory, Lecture Notes in Physics 779, Springer (2009) [doi:10.1007/978-3-642-00450-6]

  (in view of 2d CFT and string theory)

A quick review of the Knizhnik-Zamolodchikov equation in the context of an introduction to WZW model CFT is in

• Krzysztof Gawędzki, section 5.6 of: Conformal field theory: a case study (arXiv:hep-th/9904145)

A review of the definition of the Knizhnik-Zamolodchikov connection on the moduli space of genus=0 surfaces with $n$ marked points:

• Shu Oi, Kimio Ueno, section 2 of: Connection Problem of Knizhnik-Zamolodchikov Equation on Moduli Space $\mathcal{M}_{0,5}$ (arXiv:1109.0715)

In relation to hypergeometric functions and quantum groups (for more see the references below):

• Alexander Varchenko, Multidimensional hypergeometric functions and representation theory of Lie algebras and quantum groups, Adv. Ser. in Math. Phys. 21, World Sci. Publ. 1995. x+371 pp. (doi:10.1142/2467)

• V. Tarasov, Alexander Varchenko, Geometry of $q$-hypergeometric functions, quantum affine algebras and elliptic quantum groups, Astérisque 246 (1997), vi+135 pp. (arXiv:q-alg/9703044, numdam:AST_1997__246__R1_0)

On KZ-equations controlling codimension$=2$ defects in D=4 super Yang-Mills theory:

• Nikita Nekrasov, BPS/CFT correspondence V: BPZ and KZ equations from $q q$-characters [arXiv:1711.11582]

• Nikita Nekrasov, Alexander Tsymbaliuk, Surface defects in gauge theory and KZ equation, Letters in Mathematical Physics 112 28 (2022) [arXiv:2103.12611, doi:10.1007/s11005-022-01511-8]

• Saebyeok Jeong, Norton Lee, Nikita Nekrasov, Intersecting defects in gauge theory, quantum spin chains, and Knizhnik-Zamolodchikov equations, J. High Energ. Phys. 2021 120 (2021) [arXiv:2103.17186, doi:10.1007/JHEP10(2021)120]

See also

• Wikipedia, Knizhnik-Zamolodchikov equations

• I. B. Frenkel, N. Yu. Reshetikihin, Quantum affine algebras and holonomic diference equations, Comm. Math. Phys. 146 (1992), 1-60, MR94c:17024

• Valerio Toledano-Laredo, Flat connections and quantum groups, Acta Appl. Math. 73 (2002), 155-173, math.QA/0205185

• Toshitake Kohno, Conformal field theory and topology, transl. from the 1998 Japanese original by the author. Translations of Mathematical Monographs 210. Iwanami Series in Modern Mathematics. Amer. Math. Soc. 2002 $[$AMS:mmono-210$]$

• P. Etingof, N. Geer, Monodromy of trigonometric KZ equations, math.QA/0611003

• Valerio Toledano-Laredo, A Kohno-Drinfeld theorem for quantum Weyl groups, math.QA/0009181

• A. Tsuchiya, Y. Kanie, Vertex operators in conformal field theory on $\mathbf{P}^1$ and monodromy representations of braid group, Adv. Stud. Pure Math. 16, pp. 297–372 (1988); Erratum in vol. 19, 675–682

• C. Kassel, Quantum groups, Grad. Texts in Math. 155, Springer 1995

• V. Chari, , A. Pressley, A guide to quantum groups, Camb. Univ. Press 1994В.

• А. Голубева, В. П. Лексин, Алгебраическая характеризация монодромии обобщенных уравнений Книжника–Замолодчикова типа $B_n$, Монодромия в задачах алгебраической геометрии и дифференциальных уравнений, Сборник статей, Тр. МИАН, 238, Наука, М., 2002, 124–143, pdf; V. A. Golubeva, V. P. Leksin, “Algebraic Characterization of the Monodromy of Generalized Knizhnik–Zamolodchikov Equations of Bn Type”, Proc. Steklov Inst. Math., 238 (2002), 115–133

• V. A. Golubeva, V. P. Leksin, Rigidity theorems for multiparametric deformations of algebraic structures, associated with the Knizhnik-Zamolodchikov equations, Journal of Dynamical and Control Systems, 13:2 (2007), 161–171, MR2317452

• V. A. Golubeva, Integrability conditions for two–parameter Knizhnik–Zamolodchikov equations of type $B_n$ in the tensor and spinor cases, Doklady Mathematics, 79:2 (2009), 147–149

• V. G. Drinfelʹd, Quasi-Hopf algebras and Knizhnik-Zamolodchikov equations, Problems of modern quantum field theory (Alushta, 1989), 1–13, Res. Rep. Phys., Springer 1989.

• R. Rimányi, V. Tarasov, A. Varchenko, P. Zinn-Justin, Extended Joseph polynomials, quantized conformal blocks, and a $q$-Selberg type integral, arxiv/1110.2187

• E. Mukhin, V. Tarasov, A. Varchenko, KZ characteristic variety as the zero set of classical Calogero-Moser Hamiltonians, arxiv/1201.3990

On the flat vector bundles underlying the KZ-equation:

• Ivan Marin, Knizhnik-Zamolodchikov bundles are topologically trivial (arXiv:0809.3590)

A 2-dimensional higher variant (categorification) of the Knizhnik-Zamolodchikov connection via higher parallel transport:

• Lucio Simone Cirio, João Faria Martins: Categorifying the Knizhnik–Zamolodchikov connection, Differential Geometry and its Applications 30 3 (2012) 238-261 [doi:10.1016/j.difgeo.2012.03.004, arXiv:1106.0042]

Braid representations via twisted cohomology of configuration spaces

The “hypergeometric integral” construction of conformal blocks for affine Lie algebra/WZW model-2d CFTs and of more general solutions to the Knizhnik-Zamolodchikov equation, via twisted de Rham cohomology of configuration spaces of points, originates with:

• Vadim Schechtman, Alexander Varchenko, Integral representations of N-point conformal correlators in the WZW model, Max-Planck-Institut für Mathematik, (1989) Preprint MPI/89- $[$cds:1044951$]$

• Etsuro Date, Michio Jimbo, Atsushi Matsuo, Tetsuji Miwa, Hypergeometric-type integrals and the $\mathfrak{sl}(2,\mathbb{C})$-Knizhnik-Zamolodchikov equation, International Journal of Modern Physics B 04 05 (1990) 1049-1057 $[$doi:10.1142/S0217979290000528$]$

  (for $\mathfrak{sl}(2,\mathbb{C})$)

• Atsushi Matsuo, An application of Aomoto-Gelfand hypergeometric functions to the $SU(n)$ Knizhnik-Zamolodchikov equation, Communications in Mathematical Physics 134 (1990) 65–77 $[$doi:10.1007/BF02102089$]$

• Vadim Schechtman, Alexander Varchenko, Hypergeometric solutions of Knizhnik-Zamolodchikov equations, Lett. Math. Phys. 20 (1990) 279–283 $[$doi:10.1007/BF00626523$]$

• Vadim Schechtman, Alexander Varchenko, Arrangements of hyperplanes and Lie algebra homology, Inventiones mathematicae 106 1 (1991) 139-194 $[$dml:143938, pdf$]$

following precursor observations due to:

• Vladimir S. Dotsenko, Vladimir A. Fateev, Conformal algebra and multipoint correlation functions in 2D statistical models, Nuclear Physics B 240 3 (1984) 312-348 $[$doi:10.1016/0550-3213(84)90269-4$]$

• Philippe Christe, Rainald Flume, The four-point correlations of all primary operators of the $d = 2$ conformally invariant $SU(2)$ $\sigma$-model with Wess-Zumino term, Nuclear Physics B

  282 (1987) 466-494 $[$doi:10.1016/0550-3213(87)90693-6$]$

The proof that for rational levels this construction indeed yields conformal blocks is due to:

• Boris Feigin, Vadim Schechtman, Alexander Varchenko, On algebraic equations satisfied by correlators in Wess-Zumino-Witten models, Lett Math Phys 20 (1990) 291–297 $[$doi:10.1007/BF00626525$]$

• Boris Feigin, Vadim Schechtman, Alexander Varchenko, On algebraic equations satisfied by hypergeometric correlators in WZW models. I, Commun. Math. Phys. 163 (1994) 173–184 $[$doi:10.1007/BF02101739$]$

  (for $\mathfrak{sl}(2, \mathbb{C})$)

• Boris Feigin, Vadim Schechtman, Alexander Varchenko, On algebraic equations satisfied by hypergeometric correlators in WZW models. II, Comm. Math. Phys. 170 1 (1995) 219-247 [euclid:cmp/1104272957]

Review:

• Alexander Varchenko, Multidimensional Hypergeometric Functions and Representation Theory of Lie Algebras and Quantum Groups, Advanced Series in Mathematical Physics 21, World Scientific 1995 (doi:10.1142/2467)

• Ivan Cherednik, Section 8.2 of: Lectures on Knizhnik-Zamolodchikov equations and Hecke algebras, Mathematical Society of Japan Memoirs 1998 (1998) 1-96 $[$doi:10.2969/msjmemoirs/00101C010$]$

• Pavel Etingof, Igor Frenkel, Alexander Kirillov, Lecture 7 in: Lectures on Representation Theory and Knizhnik-Zamolodchikov Equations, Mathematical surveys and monographs 58, American Mathematical Society (1998) $[$ISBN:978-1-4704-1285-2, review pdf$]$

• Toshitake Kohno, Homological representations of braid groups and KZ connections, Journal of Singularities 5 (2012) 94-108 $[$doi:10.5427/jsing.2012.5g, pdf$]$

• Toshitake Kohno, Local Systems on Configuration Spaces, KZ Connections and Conformal Blocks, Acta Math Vietnam 39 (2014) 575–598 $[$doi:10.1007%2Fs40306-014-0088-6, pdf$]$

• Toshitake Kohno, Introduction to representation theory of braid groups, Peking 2018 $[$pdf, pdf$]$

  (motivation from braid representations)

See also:

• Alexander Varchenko, Asymptotic solutions to the Knizhnik-Zamolodchikov equation and crystal base, Comm. Math. Phys. 171 1 (1995) 99-137 $[$arXiv:hep-th/9403102, doi:10.1007/BF02103772$]$

• Edward Frenkel, David Ben-Zvi, Section 14.3 in: Vertex Algebras and Algebraic Curves, Mathematical Surveys and Monographs 88, AMS 2004 $[$ISBN:978-1-4704-1315-6, web$]$

This “hypergeometric” construction uses results on the twisted de Rham cohomology of configuration spaces of points due to:

• Peter Orlik, Louis Solomon, Combinatorics and topology of complements of hyperplanes, Invent Math 56 (1980) 167–189 $[$doi:10.1007/BF01392549$]$

• Kazuhiko Aomoto, Gauss-Manin connection of integral of difference products, J. Math. Soc. Japan 39 2 (1987) 191-208 $[$doi:10.2969/jmsj/03920191$]$

• Hélène Esnault, Vadim Schechtman, Eckart Viehweg, Cohomology of local systems on the complement of hyperplanes, Inventiones mathematicae 109.1 (1992) 557-561 $[$pdf$]$

• Vadim Schechtman, H. Terao, Alexander Varchenko, Local systems over complements of hyperplanes and the Kac-Kazhdan conditions for singular vectors, Journal of Pure and Applied Algebra 100 1–3 (1995) 93-102 $[$arXiv:hep-th/9411083, doi:10.1016/0022-4049(95)00014-N$]$

also:

• Peter Orlik, Hypergeometric integrals and arrangements, Journal of Computational and Applied Mathematics 105 (1999) 417–424 $[$doi:10.1016/S0377-0427(99)00036-9, pdf$]$

• Daniel C. Cohen, Peter Orlik, Arrangements and local systems, Math. Res. Lett. 7 (2000) 299-316 $[$arXiv:math/9907117, doi:10.4310/MRL.2000.v7.n3.a5$]$

reviewed in:

• Yukihito Kawahara, The twisted de Rham cohomology for basic constructions of hyperplane arrangements and its applications, Hokkaido Math. J. 34 2 (2005) 489-505 $[$doi:10.14492/hokmj/1285766233$]$

Discussion for the special case of level$=0$ (cf. at logarithmic CFT – Examples):

• Fedor A. Smirnov, Remarks on deformed and undeformed Knizhnik-Zamolodchikov equations, $[$arXiv:hep-th/9210051$]$

• Fedor A. Smirnov, Form factors, deformed Knizhnik-Zamolodchikov equations and finite-gap integration, Communications in Mathematical Physics 155 (1993) 459–487 $[$doi:10.1007/BF02096723, arXiv:hep-th/9210052$]$

• S. Pakuliak, A. Perelomov, Relation Between Hyperelliptic Integrals, Mod. Phys. Lett. 9 19 (1994) 1791-1798 $[$doi:10.1142/S0217732394001647$]$

Interpretation of the hypergeometric construction as happening in twisted equivariant differential K-theory, showing that the K-theory classification of D-brane charge and the K-theory classification of topological phases of matter both reflect braid group representations as expected for defect branes and for anyons/topological order, respectively:

• Hisham Sati, Urs Schreiber, Anyonic defect branes in TED-K-theory $[$arXiv:2203.11838$]$